Difference between revisions of "Lecture 1. - Assignment"
(→Knowledge needed to solve the problem) |
(→Knowledge needed to solve the problem) |
||
| (2 intermediate revisions by the same user not shown) | |||
| Line 32: | Line 32: | ||
YouTube channel for [https://www.youtube.com/channel/UC7WzdNsW6Si-8e96mSluPPg Agros2D].<br/> | YouTube channel for [https://www.youtube.com/channel/UC7WzdNsW6Si-8e96mSluPPg Agros2D].<br/> | ||
| − | There are also a lot of videos on YouTube for using FEMM. | + | There are also a lot of videos on YouTube for using FEMM. For example [https://www.youtube.com/watch?v=1BKCkvr83j4 FEMM Tutorial] |
== The Problem == | == The Problem == | ||
| Line 86: | Line 86: | ||
=== Knowledge needed to solve the problem === | === Knowledge needed to solve the problem === | ||
The problem solution with | The problem solution with | ||
| − | * [FEMM] | + | * [https://youtu.be/bRMxkaXJnwQ FEMM] |
| − | * [Agros2D] | + | * [https://youtu.be/uO-jPNcDfIM Agros2D] |
== References == | == References == | ||
{{Reflist}} | {{Reflist}} | ||
Latest revision as of 10:59, 6 April 2021
|
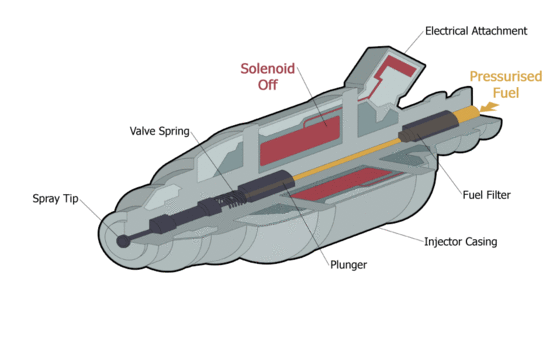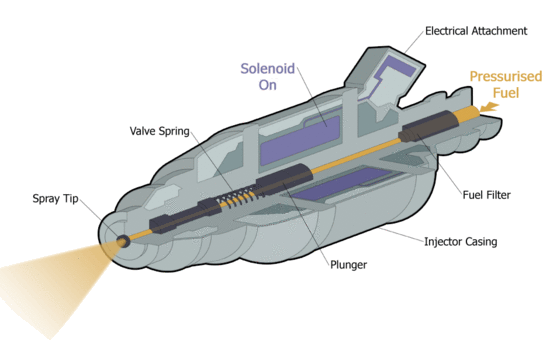
Fuel Injection Solenoid | |
| The fuel injection in operation. [1] | The magnetic flux density vectors in the plunger after switch on the solenoid. |
|
Instructor
|
Teaching Assistants:
|
Contents
[hide]Purpose of the Assignment
The student will learn the main steps of the finite element method, such as creating the model (creating or importing geometry), specifying material parameters, boundary conditions, and excitation through simulation of the electromagnetic part of the fuel injector.
Knowledge needed to solve the problem
- The steps of the finite element method;
- Theoretical knowledge of the static magnetic field (for defining materials, for excitation);
- Knowledge of the basics of FEMM and Agros2D.
YouTube channel for Agros2D.
There are also a lot of videos on YouTube for using FEMM. For example FEMM TutorialThe Problem
The problem (if we neglect the transient state occurring at the switch on) can be considered as an axisymmetric static magnetic problem. The effect of the movement of the iron core (valve opening/closing) is also examined accordingly, i.e. each position is an individual simulation.
The height of the cylindrical core is 14mm and the radii is 3.6mm. Dimensions of the coil: height is 20mm, inner radii is 4mm and outer radii is 10mm.
The materials:
- Core/Vasmag: Steel with nonlinear B-H curve (Steel1008);
- Coil/Tekercs: Copper (μr=1.0) and the number of turns is 2000. The current is 0.2 A;
- Region: Air (μr=1.0).
The task is to determine the inductance of the solenoid valve and the force acting on the iron core. As a first step, it is recommended to validate the simulation with the values given in the table when the iron core is in the middle of the solenoid. Then it is worth examining the two quantities as a function of the position of the iron core.
Inductance Calculation
The most widely used method for determining inductance in finite element analysis is based on magnetic energy. From the magnetic energy, the inductance L can be determined by the following formula:
- L=2⋅WmI2
where Wm the magnetic energy of the whole problem and I is the current (in this case 0.2A).
Force Calculation
The force acting on the iron core can also be determined by surface and line integration. In the case of surface integration, the core should be selected. However, in both cases, Maxwell's stress tensor (FEMM - Force via Weighted Stress Tensor / Agros2D - Maxwell force - y) used for integration.
Evaluation of Results
Here you can plot the field quantities (magnetic flux density, magnetic vector potential, etc.) and checked the value of the inductance of the coil and the acting force on the iron core. The following values were obtained for these quantities, the arrangement according to the cross-sectional view being 0 mm position.
The results of the simulation for validation. Software Maxwell 2D Maxwell 3D FEMM Agros2D Inductance [mH] 51.911 51.812 51.821 51.89 Force [μN] 88.603 63.29 74.53 57.45 In addition, it is possible to parameterize the geometry or most of the simulation parameters (position, size, current, number, ...) via script (FEMM - LUA script, Agro2D - Python). The result of a parameterized simulation is shown in the figure where the parameter is the position of the iron core. As a result, the modification of the parameter and the evaluation of results are automatic.
Knowledge needed to solve the problem
The problem solution with
References